The Tamilian Statement of the Pythagoras Theorem

Pythagoras Theorem, as every one would have studied in their high school mathematics, is defined as follows

"In any right triangle, the area of the square whose side is the hypotenuse (the side of the triangle opposite the right angle) is equal to the sum of the areas of the squares of the other two sides."

so the hypotenuse can be calculated as h = (a² + b²)½, that is square root of (a² + b²). But there is an amazing theorem in Tamil, rather a statement to find out the hypotenuse without the use of square-roots. This was stated, by Bodhayanar or Buddhayana, even before the time of Pythagoras. The statement goes like this

"Odum Neelam Thanai Ore Ettu
Kooru thaaki Koorilae Ondrai
Thalli Kundrathil Paadhiyai Saerthal
Varuvathu Karnam Thane"

which means split the base (Neelam) into 8 equal parts (Kooru) and subtract 1 part from it and add half the height (Kundru) which gives the hypotenuse (Karnam). So lets work this out with an example. Consider a right triangle, of dimensions base 8 units and height 6 units so according to the pythagoras theorem the hypotenuse is square root of (64 + 36) which is 10. Now lets try the same with the above theorem

Divide the base into 8 equal parts so in this case each part is 1 unit

Subtract 1 part from the 8 parts so the remaining is 7 units ----- (1)

Add half the height which is 1/2 of 6 = 3 units -----(2)

Now adding (1) and (2) gives the total 10 units which is the hypotenuse of the triangle.
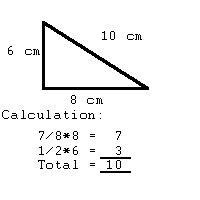Amazing isn't it? Also if you notice there is no use of any square roots, but simple basic arithmetic addition, subtraction, multiplication and division. The only condition for this to work is that the base has to be greater than the height. So we can summarize the above as follows. If x, y are the base and height of a right triangle respectively then the hypotenuse

z = 7/8*x + 1/2*y where x > y

This works 100% accurately for all integral pythagorean triplets (i.e, the right triangles with dimensions that are round numbers like [3,4,5], [8,6,10], [5,12,13] etc) for the others it works with 95% to 99% accuracy

I don't have any rigorous mathematical proof to it, but it works!

More to come, until then...